2. The history of Analytical Geometry

2.1. René Descartes

“I am thinking, therefore I exist.” or “I think, therefore I am.” (Latin: Cogito ergo sum) René Descartes: Discours de la Méthode, 1637 “Each problem that I solved became a rule which served afterwards to solve other problems.” René Descartes: Discours de la Méthode, 1637

René Descartes (1596-1650) is generally regarded as the father of Analytical Geometry . His name in Latin is Renatius Cartesius — so you can see that our terminology “Cartesian plane” and “Cartesian coordinate system” are derived from his name! Analytical Geometry is also often called Cartesian Geometry or Coordinate geometry.

Descartes is also generally regarded as the father of modern philosophy. His life spanned one of the greatest intellectual periods in the history of all civilization. To mention only a few of the giants: Fermat and Pascal were his contemporaries in mathematics. Shakespeare died when Descartes was twenty, Descartes outlived Galileo by eight years, and Newton was eight when Descartes died. Descartes is so famous that the town in France where he was born — La Haye — has been renamed to Descartes. His face has been on many stamps throughout the world.

Descartes believed that a system of knowledge should start from first principles and proceed mathematically to a series of deductions, reducing physics to mathematics. In his Discours de la Méthode (1637) — the full title was “Discourse on the Method of Rightly Conducting the Reason and Seeking Truth in the Sciences” — he advocated the systematic doubting of knowledge, believing as Plato that sense perception and reason deceive us and that man cannot have real knowledge of nature. The only thing that he believed he could be certain of was that he was doubting, leading to his famous phrase "Cogito ergo sum", (I think, therefore I am). From this one phrase, he derived the rest of his philosophy.

Descartes formulates the following principles for the reasoning process:

• accept nothing as true except that which you recognize as clearly such;
• divide each difficulty that you meet into manageable pieces;
• proceed in your thinking, stage by stage, from the simple to the complex;
• review your thinking carefully to ensure that nothing has been omitted.

Descartes showed that if a geometric construction requires in its analytic form nothing but addition, subtraction, multiplication, division, and the extraction of square roots, then it can be achieved with ruler and compass. These arithmetic operations are to be applied to the two coordinates of each point given by the construction problem. Conversely if it can be achieved with ruler and compass, then when represented analytically all points involved in the construction will have coordinates that can be obtained from those of the points initially given by these five arithmetic operations. The results may be very complicated, for example, if (a; b) and (c; d) are two of the points given, one new coordinate might be

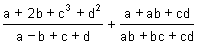
This point reached, we can now concentrate almost entirely on the algebra!